The global financial crises of 2007-2009 has shattered our confidence in economic theory. After more than twenty years of intense academic research in banking economics, we realize that we still do not know much. For example, economists continue to often rely on the efficient market hypothesis, and on the Modigliani Miller theorem, which we know are based on strong and unrealistic assumptions.
Policy makers need more realistic models to guide their decisions on financial stability, in particular with respect to the appropriate level of capital requirements for banks, that is the focus of this note (albeit the line of reasoning that I will propose has a broader reach).
Straight after the crisis, the observation of the current limits of economists’ models and academic research was a fantastic opportunity for the economic profession to try to sit together and find a consensus on new paradigms that will help policy makers. But, in fact, on the specific question of bank capital, the opposite has happened, with the polarization of the debate and currently two groups that refuse any dialog.
On the one hand, the group led by Anat Admati and Martin Hellwig recommends a minimum capital ratio of 30%, as reported in their new book “The Bankers’ New Clothes: What’s Wrong with Banking and What to Do about It”. Although they are in favour of higher capital for banks than it is currently the case, and so am I, it is disappointing to notice that there is not a single quantitative argument in the book that justifies such a figure. These authors do not offer scientific arguments nor quantitative analysis in support of their thesis and, still, they are currently backed by very influential and prestigious people such as Roger Myerson, Mervyn King and the journalist Martin Wolf.
On the other hand, the group that we can call “the Business School economists”, such as Gary Gorton, and more recently, Harry DeAngelo and René Stulz, think that high leverage is desirable for banks. Their reasoning is that banks have to provide liquidity to investors and if one forces banks to have too much equity, then they will provide too little liquidity. We do need a model to properly address and support these types of claims.
The current dismal state of affairs is that instead of having worked hard to develop new models, those two groups have kept their ideological positions.
The urgency of a different approach can be noticed by observing, for example, the evolution of bank capital ratios in the US from the nineteenth century until today. Clearly, this shows a downward trend: in the 1840s the capital ratio was higher than 50%, while in the recent years it went down to less than 10%.

Indeed, the presence of a downward trend in capital ratios does not prove anything, because many other things changed over this long time span. Blaming on the decline of capital ratios without further analysis would be as claiming that the downward trend of the world record for the 100 meters male sprinter in the last 70 years is a dangerous path and we should rather slow down.
Of course many serious empiricists have done empirical studies on the correlation between banks’ leverage, growth, systemic risk. And they almost unanimously suggest that there is a trade-off. On the one hand, higher capital ratios imposed to banks or, similarly, less leverage or a tighter limit on banks’ loan supply, improve systemic stability, because allow banks to absorb larger losses. On the other hand, these measures negatively affect growth, because they restrict lending to the economy.
However, this association between banks’ leverage, growth and systemic risk is just a simple correlation and, as many have argued, cannot be interpreted in terms of causality. In this respect, Steve Ongena from Zurich University and his co-authors have convincingly emphasized that to disentangle demand and supply effects within a proper structural model one needs microeconomic data on single bank relationships, which are often confidential, rare and difficult to investigate.
These intrinsic limitations show why theorists can have a fundamental impact in our understanding. To do proper economic research one needs both data and theory, but if a proper structural model to identify causality links is not available, the best one can do is to interpret correlations with the help of theoretical models. Consider for example, the evolution of bank capital ratios in the US in the last 90 years (Figure 2). Plotting the capital ratio against the spread between loan and deposit rates or against the interest margin, one cannot identify a clear correlation, or any kind of statistical regularity. This is normal. Because both variables are endogenous and vary over time, in order to understand and interpret these empirical observations, one needs a model that explains these movements together.
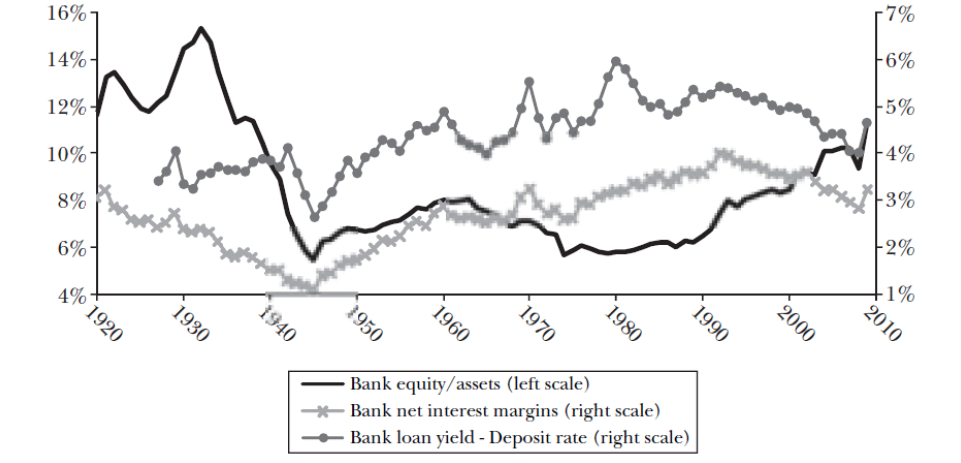
Going back to the main theme under analysis, we are therefore to ask ourselves what is the role of bank capital. If we believe in the Modigliani and Miller theorem, the dimension of a bank’s capital should not matter, because the structure of the liabilities of the bank is irrelevant within the framework of this theorem. But clearly this is not a very satisfactory answer, and we need to go further in our research; in particular, we need to account for the role of frictions in our paradigm of analysis.
Before further elaborating, it is important to clarify the precise and specific role of capital that is of interest in the present discussion. Most academics, including Jean Tirole, have emphasized the role of bank capital in providing incentives for bankers. It is the idea of having some “skin in the game”: if you have a lot to lose in the bank that you have financed, then you will be more careful about the risk that your manager takes. Although this is the leading paradigm among economists, and it was at the center of the discussion around the first Basel pillar, it turned out that there was a major misunderstanding with regulators. What regulators have in mind about the role of capital is completely different and has nothing to do with incentives. For regulators, bank capital is a buffer against losses, that protects depositors and that allows for some precious extra-time in the resolution in failing of banks. This is especially true for the so called SIFIs (Systemically Important Financial Institutions): more capital allows to absorb losses and to resolve a failing institution in a proper and ordered fashion. Clearly, this has nothing to do with incentives which instead are affected by “inside equity”: i.e., the equity that is held by the top management. This capital, which also includes the remuneration package of managers, has an impact on their risk taking behaviour – incidentally, it is useful to notice that regulators have finally considered the possibility to regulate bankers’ compensation. However, if we look at the regulation of banks’ total capital, this pertains not only to the tiny part that is held by managers, but also, and mainly, to the very large part that is held by shareholders that have no say on the decisions of the bankers. Regulation of banks’ capital does not refer to managers’ incentives, which should be dealt with by regulation of compensation. It has instead to do with loss absorbing capacity.
We are now at a crossroad. Economists, especially macro and monetary economists, have played a very important role in helping out central banks for monetary policy decisions. The development of the DSGE models – Keynesian type models with which it is possible to try to understand the impact of monetary policy interest rates on short term employment and growth – is a fantastic, even though sometimes criticized, success of our profession. For monetary policy we have models that one can simulate and that can be used in order to understand the consequences of changing short term interest rates. However, those models were designed for monetary policy, which has a short-time horizon and has certain objectives in terms of inflation and, to some extent, output; but that has nothing to do with financial stability. Financial stability is a long run objective and then it requires different models.
Although many economists have tried to introduce banks and financial frictions into DSGE models, these models are too complicated with so many interacting “blocks” (to reproduce data in the short term), that by adding another layer of complexity they lose transparency and the possibility to interpret the results. This is not a secondary issue, because this lack of transparency affects the accountability of policy decisions. In democracy, and for the sake of our economies, it is important that policy makers and people that take decisions on bank capital ratios, such as the Basel committee, are accountable for their choices. Why is that, until recently, we only required a 4% of capital and now apparently many people think that the appropriate level is 25%-30%? If a complex model and its complex numerical simulations deliver a certain desirable outcome by increasing capital ratios, why would we believe this outcome? We do need models and, in particular, simple and transparent models, so that policy makers can explain and justify their decisions, avoiding to take them on the basis of ideology.
Fortunately, the quest for these simple models is something that several people are working on at the moment, and new interesting ideas are emerging, emphasizing the role of banks in the provision of liquidity, as suggested in DeAngelo and Stulz (2013) and Gorton (2013).
Indeed, banks provide liquidity and collect deposits, and also provide financing to firms. So, the crucial technology of a bank is to transform riskless deposits into risky assets. In this respect, equity is exactly what one needs to buffer the associated possible losses, so that depositors can be sure that in any circumstance they will get their money back. Hence, liquidity implies completely risk free deposits. There are several new investigations emphasizing these dimensions. For example, Gennaioli, Sheifler and Vishny (2012 and 2015) have models in which depositors are infinitely risk averse, and that’s the way to capture the notion that, if there is a risk in your deposit, then you might be unable to write a check on it. Similarly, Stein (2013) has a model in which bank deposits provide utility per-se, because of the liquidity of those deposits. Even Hellwig himself (2015) has a recent paper on this topic. He shows that the argument of DeAngelo and Stulz (2013) – according to which if one has too much liquidity there are then too little deposits or, in other words, that equity and deposits are substitutes – is completely wrong as soon as there is risk on the asset side. In this case, in fact, in order to provide safe deposits, a bank needs something to buffer the losses, and this is precisely capital.
But the main problem with these models is that they are static and therefore cannot be brought to the data, or even calibrated. This is why we need to explore this ideas in a dynamic setup. A few models have been recently developed in discrete time (e.g., De Nicolo, Gamba e Lucchetta, 2013) and also in continuous time, such as the very influential models by Brunnermeier and Sannikov (2014) and by He and Krishnamurhty (2012 and 2013). But these papers are quite complex, and as stated above, we need simple and transparent models to make policy decisions accountable.
This is the line of research I am currently working on, for example in Klimenko, Pfeil and Rochet (2015), “Bank Capital and Aggregate Credit”. This is a general equilibrium model with frictions: the general equilibrium dimension is needed to understand the feedback loops, frictions are needed to eliminate the indifference results of the Modigliani and Miller theorem. This model is in the spirit of Brunnermeier and Sannikov (2014), but it is much simpler because we used a Occam’s razor approach to eliminate any ingredient that was not strictly needed to understand the role of bank capital. Although it is not the perfect model, it nevertheless provides an understanding of the impact of increasing capital requirements, both in the short term and in the long term. Importantly, we can notice that the short term impact is very different from the long term impact, and this is precisely why we need a dynamic model. The conclusion of this specific study is that, although the two short-run dimensions (stability vs. growth) are conflicting, in the long run, if the capital ratio is not unreasonably high, there is the possibility to reconcile them. In fact, it is possible to find an equilibrium where the economy is more stable and it grows at a higher rate, because people have a higher trust in the stability of the banking sector.
Developing a new class of macro models will be fundamental in the next years to understand the impact of financial stability decisions, because we do not want to accept purely ideological statements such as that bank capital should be 30% – or instead 4% – without knowing why this may be the case. And we do not want regulators deciding on the basis of pure authority arguments either. Economists will need to provide models and figures that come from a rigorous and scientific analysis. With this respect, simple models are valuable in that they enlighten the short term and long term impact of increasing capital requirements.
References
Admati, A. and Hellwig, M., 2013. The Bankers’ New Clothes: What’s Wrong With Banking and What to Do about it? Princeton, Princeton University Press.
Baker, M. and Wurgler, J., 2013. Do Strict Capital Requirements Raise the Cost of Capital? Banking Regulation and the Low Risk Anomaly. NBER Working Papers 19018, National Bureau of Economic Research.
Brunnermeier, M. K., and Sannikov, Y., 2014. A Macroeconomic Model with a Financial Sector. American Economic Review, 104(2): 379-421.
Corbae, D. and D’Erasmo P., 2014. Capital requirements in a quantitative model of banking industry dynamics. Working Papers 14-13, Federal Reserve Bank of Philadelphia.
De Nicolò, G., Gamba, A. and Lucchetta M., 2014. Microprudential Regulation in a Dynamic Model of Banking. The Review of Financial Studies, ISSN: 0893-9454
DeAngelo, H., and Stulz, R., 2013. Why High Leverage is Optimal for Banks. OSU Working Paper.
De Nicolò, G., 2015. Revisiting the impact of bank capital requirements on lending and real activity. mimeo IMF, June.
Egenau, J., 2014. Capital Requirements, Risk Choice, and Liquidity Provision in a Business Cycle Model. Harvard Business School Working Paper, No. 15-072.
Gennaioli, N., Shleifer, A. and Vishny, R. W., 2015. Neglected Risks: The Psychology of Financial Crises. American Economic Review 105 (5): 310-314.
Gennaioli, N., Shleifer, A. and Vishny, R. W., 2012. Neglected Risks, Financial Innovation and Financial Fragility. Journal of Financial Economics 104 (3): 452-468.
Gorton, G., 2013. The supply and demand for safe assets. National Bureau of Economic Research.
He, Z. and Krishnamurthy, A., 2013. Intermediary Asset Pricing. American Economic Review 103(2), pp. 732-770.
He, Z. and Krishnamurthy, A., 2012. A Model of Capital and Crises. Review of Economic Studies 79(2): pp. 735-777.
Hellwig, M., 2015. Liquidity Provision and Equity Funding of Banks. Working Paper.
Jimenez, G., Ongena, S., Peydró, J-L. and Saurina, J., 2012. Macroprudential Policy, Countercyclical Bank Capital Buffers and Credit Supply: Evidence from the Spanish Dynamic Provisioning Experiments. Working Papers 628, Barcelona Graduate School of Economics.
Klimenko, N., Pfeil, S. and Rochet, J-C., 2015. Bank Capital and Aggregate Credit. Working Paper.
Martinez-Miera, D. and Suarez, J., 2014. Banks’ endogenous systemic risk taking. Working Paper.
Miles, D., Yang, J., and Marcheggiano, G., 2013. Optimal Bank Capital. Economic Journal, Royal Economic Society, vol. 123(567), pages 1-37, 03.
Nguyen, T. T., 2014. Bank Capital Requirements: A Quantitative Analysis. Working Paper.
Phelan, G., 2014. Financial Intermediation, Leverage, and Macroeconomic Instability. Mimeo, Yale University.
Stein, J. C., 2013. Monetary Policy as Financial Stability Regulation. The Quarterly Journal of Economics (2012) 127 (1): 57-95.
Stein, J., Hanson, S. and Kashyap, A. K., 2011. A Macroprudential Approach to Financial Regulation. Journal of Economic Perspective 25 (1): 3-28.